Nuclear binding energy is the energy required to break up the nucleus into its separate nucleons OR this can be expressed as the energy released when the nucleus is formed from separate nucleons.

Binding energy is equal to the decrease in potential nuclear energy of the nucleons when they come together. This is equivalent to the work done on the nucleons by the strong nuclear force.

Binding energy is the energy associated with the strong force that holds the nucleons together.

The mass of a nucleus is less than the mass of the individual nucleons that make up that nucleus. The mass difference (m) between the two is equivalent to the binding energy of the nucleus.

The relationship between binding energy and mass difference is given by Einstein's equation:

E b = m c²

On the AQA board data sheet there is a conversion factor from mass (u) to energy in MeV - this saves you converting the mass into kilogram and then using E = mc2 to work out the energy in jouls and then convert the energy in joules to MeV).

m is difference between mass of nucleus and total mass of nucleons

m = Zm_p + (A – Z)m_n – m _nucleus

where:

Binding Energy Per Nucleon

If we know the binding energy in a nucleus, and the number of nucleons, we can work out the binding energy per nucleon, which is the average energy needed to remove each nucleon.

The higher the binding energy per nucleon, the more stable is the nucleus.

We can plot a graph of binding energy per nucleon against nucleon number and it gives a smooth curve - with one remarkable anomaly - helium (⁴He) the binding energy per nucleon is:

Binding energy per nucleon of helium = 28.38 MeV / 4 = 7.1 MeV

This is not where we would expact on the general curve.

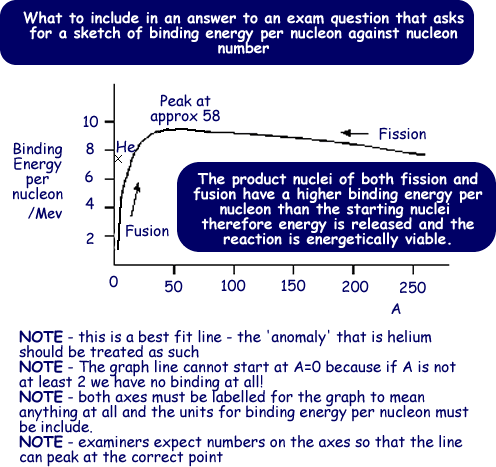
Iron has the one of the highest binding energy per nucleon values - so the graph peaks around A=58 - here we find the most stable nuclei.

If we look at large nuclei (much greater than iron), we find that the further to the right (greater nucleon number) the less stable the nuclei. This is because the binding energy per nucleon is getting less. These nuclei undergo fission and split to produce products with higher binding energy per nucleaon values - more stable products.

If we look at nuclei of much smaller mass than iron we find they have a lower binding energy per nucleon. Therefore when these fuse to produce a heavier nucleus it is more stable - fusion can be shown to be energetically viable from the above graph. The product nucleus has a higher binding energy pernucleon than the two that fuse to form it. It is therefore more stable than its constituents.